You're reading the public-facing archive of the Category Theory Zulip server.
To join the server you need an invite. Anybody can get an invite by contacting Matteo Capucci at name dot surname at gmail dot com.
For all things related to this archive refer to the same person.
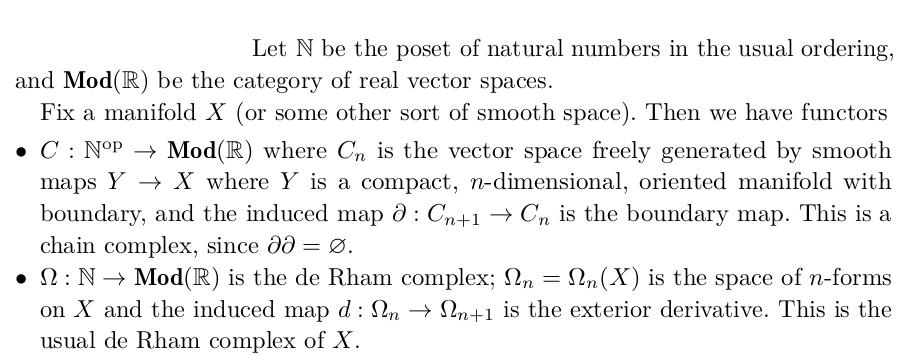
Tim Hosgood (Apr 01 2020 at 19:36):There's a nice little story about how you can understand Stokes' theorem as a coend (see e.g. https://twitter.com/ququ7/status/1169048827703287814), but I can't figure out how it's really a co_end_ and not just a co_wedge_, i.e. what does the universality condition of the coend tell you in all this?
Fun fact: Stokes' theorem is a result about coends. (credits to Tim Campion for having told me that a few years back!) https://twitter.com/ququ7/status/1169048827703287814/photo/1
- Fo Uche (@ququ7)
Amar Hadzihasanovic (Apr 02 2020 at 16:05):The “coend” part is the “Bonus point” part of what Fosco wrote, isn't it?
The existence of the Stokes cowedge induces a universal map from the coend of to the constant functor. I have not tried to solve the exercise to see what it amounts to, though.
Tim Hosgood (Apr 02 2020 at 17:58):ah, that would make sense! maybe i'll try to have a think about this bonus exercise then